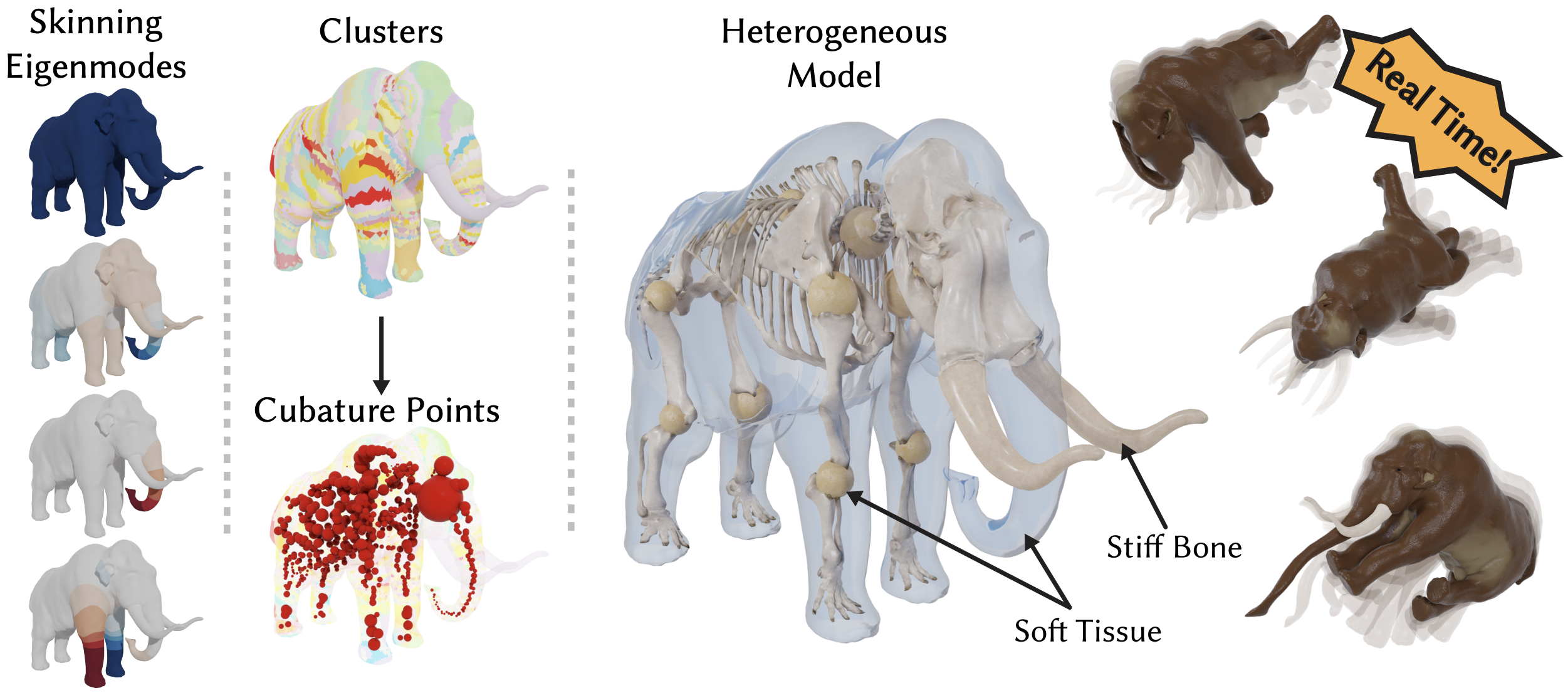

Real-time elastodynamic solvers are well-suited for the rapid simulation of ho mogeneous elastic materials, with high-rates generally enabled by aggressive early termination of timestep solves. Unfortunately, the introduction of strong domain heterogeneities can make these solvers slow to converge. Stopping the solve short creates visible damping artifacts and rotational errors. To address these challenges we develop a reduced mixed finite element solver that pre serves rich rotational motion, even at low-iteration regimes. Specifically, this solver augments time-step solve optimizations with auxillary stretch degrees of freedom at mesh elements, and maintains consistency with the primary positional degrees of freedoms at mesh nodes via explicit constraints. We make use of a Skinning Eigenmode subspace for our positional degrees of freedom. We accelerate integration of non-linear elastic energies with a cubature approx imation, placing stretch degrees of freedom at cubature points. Across a wide range of examples we demonstrate that this subspace is particularly well suited for heterogeneous material simulation. Our resulting method is a subspace mixed finite element method completely decoupled from the resolution of the mesh that is well-suited for real-time simulation of heterogeneous domains.
This work is funded in part by NSERC Discovery (RGPIN-2017-05524), Connaught Fund (503114), CFI-JELF Fund, Accelerator (RGPAS-2017-507909), the Canada Research Chairs Program, and the Ontario Early Researchers Award.